基于变径基圆渐开线涡旋压缩机的几何模型及优化研究

doi:10.16183/j.cnki.jsjtu.2022.129

基于变径基圆渐开线涡旋压缩机的几何模型及优化研究

彭斌^,, 刘慧鑫, 陶耀辉

兰州理工大学机电工程学院,兰州 730050

Geometrical Model and Optimization of Scroll Compressor Based on Involute of Circle with Variable Radii

PENG Bin^,, LIU Huixin, TAO Yaohui

School of Mechanical and Electrical Engineering, Lanzhou University of Technology, Lanzhou 730050, China

责任编辑: 王一凡

收稿日期: 2022-04-27 修回日期: 2022-06-6 接受日期: 2022-06-17

基金资助:

国家自然科学基金资助项目(51675254)
国家自然科学基金资助项目(51966009)
国家重点研发计划“科技助力经济2020”重点专项(SQ2020YFF0420989)
兰州市人才创新创业项目(2020-RC-23)
甘肃省科技计划项目(20YF8GA057)
甘肃省教育厅优秀研究生“创新之星”项目(2021CXZX-442)

Received: 2022-04-27 Revised: 2022-06-6 Accepted: 2022-06-17

作者简介 About authors

彭斌(1976-),教授,博士生导师,从事涡旋机械、低温余热发电技术研究;E-mail:pengb2000@163.com.

摘要

针对现有变径基圆涡旋压缩机几何模型的定量分析及优化研究不够完善的问题,给出了变径基圆涡旋压缩机从吸气过程开始直到排气过程结束的完整工作腔容积变化规律.比较了格林定理法、图解法及法向等距法的容积计算差异性,分析了产生计算误差的原因,验证了几何模型的正确性.推导了齿面积利用率的计算公式,构建了行程容积及齿面积利用率的灵敏度模型,得出基圆半径变化系数及内侧型线发生角的影响尤为显著的结论.建立了一种考虑非线性约束且以行程容积与齿面积利用率为优化目标的多目标优化分析模型.结果表明:多目标遗传算法(NSGA-II)比多目标粒子群(MOPSO)算法综合性能更佳,且相较于优化前,某款汽车空调用变径基圆涡旋压缩机的行程容积提升了6.5%,齿面积利用率降低了4.27%.该研究能为变径基圆涡旋压缩机的优化设计及定量研究提供进一步的理论参考.

关键词： 涡旋压缩机; 变径基圆涡旋型线; 几何模型; 多目标优化; 算法对比

Abstract

Considering the problems that quantitative analysis and optimization of the geometrical model of scroll compressor created from involute of circle with variable radii are incomplete, the changing trend of the working chamber volume from the beginning of the suction process to the end of the discharge process is given. The volume calculation differences of Green’s theorem method, the graphical method, and the normal isometric method are compared, the reasons for calculation errors are analyzed, and the correctness of the geometrical model is verified. The calculation formula of teeth area utilization rate is deduced, and the sensitivity models of suction volume and teeth area utilization rate are constructed. It is concluded that the influence of the base circle radius variation coefficient and the occurrence angle of the inner profile are particularly significant. A multi-objective optimization analysis model considering nonlinear constraints and taking the suction volume and teeth area utilization rate as the optimization goals is established. The results show that the multi-objective genetic algorithm NSGA-II has a better comprehensive performance than the multi-objective particle swarm algorithm MOPSO and the suction volume of the scroll compressor created from the involute of circle with variable radii for an automobile air conditioner has been increased by 6.5% and the teeth area utilization rate has been reduced by 4.27%. This paper can provide further theoretical reference for the optimal design and quantitative research of the scroll compressor constructed from involute of circle with variable radii.

Keywords： scroll compressor; involute of circle with variable radii; geometrical model; multi-objective optimization; algorithm comparison

PDF (3426KB) 元数据多维度评价相关文章导出 EndNote| Ris| Bibtex 收藏本文

本文引用格式

彭斌, 刘慧鑫, 陶耀辉. 基于变径基圆渐开线涡旋压缩机的几何模型及优化研究[J]. 上海交通大学学报, 2023, 57(8): 1046-1054 doi:10.16183/j.cnki.jsjtu.2022.129

PENG Bin, LIU Huixin, TAO Yaohui. Geometrical Model and Optimization of Scroll Compressor Based on Involute of Circle with Variable Radii[J]. Journal of Shanghai Jiaotong University, 2023, 57(8): 1046-1054 doi:10.16183/j.cnki.jsjtu.2022.129

涡旋压缩机具有结构简单、噪声低、效率高等优点,广泛应用于制冷、医疗、食品等行业^[1].传统由基圆渐开线构成的涡旋压缩机在几何理论、热动力学模型及优化等方面的研究已经趋于成熟^[2].而变径基圆涡旋压缩机较高的齿头强度、较低的齿高、较小的涡旋齿质量使得其具有更好的综合性能^[3-4].

目前,对变径基圆涡旋压缩机的研究主要集中在型线设计及几何模型推导层面,田亚永^[5]利用微分几何共轭曲面理论,证明了变径基圆渐开线作为涡旋型线的可行性;丁佳男等^[6]推导了渐开线发生角是否在X轴起始位置的两种变基圆涡旋型线的双圆弧齿头修正几何模型;王吉岱等^[7]构建了变基圆涡旋膨胀机的几何模型并推导了各膨胀腔的容积计算公式;唐景春等^[8]推导了变径基圆型线齿头双圆弧加直线修正几何模型,研究了修正角度对修正齿轴向投影面积及内压比的影响.

对涡旋压缩机的优化主要集中在几何参数的研究,采用的方法主要为线性加权法、pareto支配法.陈进等^[9]首次提出了利用多目标遗传算法优化涡旋型线参数的方法;彭斌等^[10]推导了单涡圈双圆弧及双圆弧加直线修正齿头的几何模型,借助多目标遗传算法对各参数进行了优化分析;Peng等^[11]利用改进的遗传算法对低压比的双涡圈涡旋压缩机的比功率及型线参数进行了优化选取;刘涛等^[12]基于自适应的NSGA-II算法,以轴向力与内容积比为优化目标,对变截面涡旋压缩机性能进行了优化研究.

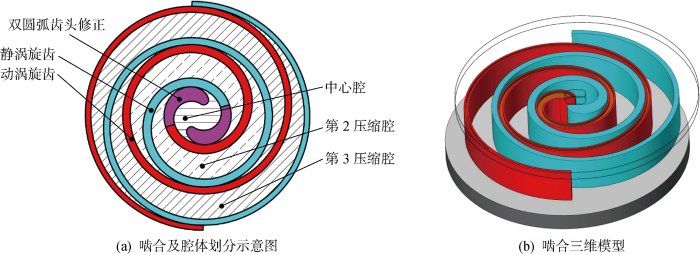
以上对涡旋压缩机的研究主要集中在等壁厚圆渐开线涡旋类型且关于变径基圆涡旋压缩机几何模型的研究,存在着模型不完善、不精确等问题.同时对变径基圆涡旋压缩机的优化研究考虑的约束较少^[13].因此,本文在完善变径基圆涡旋压缩机几何模型的基础上,尝试构建一种考虑约束条件的多目标优化分析模型,为变径基圆涡旋压缩机的优化研究及定量分析提供进一步的理论参考.

1 变径基圆涡旋压缩机的几何模型

1.1 变径基圆涡旋压缩机涡旋齿的几何模型

变径基圆涡旋压缩机涡旋齿结构是由相位差为π的动静涡旋齿构成,其基本几何结构参数如表1所示,根据法向等距原理^[14]推导其动涡旋齿型线方程如下.

表1 基本几何结构参数

Tab.1 Basic geometric parameters

参数	符号	取值
初始基圆半径	a₀/mm	3.675
基圆半径变化系数	δ₀/(mm·rad^-1)	-0.05
离散的多方指数	δ₁	1
回转半径	R_ob/mm	4.361 7
内侧型线发生角	α_in/rad	1.430 44
外侧型线发生角	α_ou/rad	0
内外侧型线展角	φ_in, φ_ou/rad	—
修正展角	Φ/rad	0.6π
终端渐开线展角	φ_e/rad	6π

新窗口打开| 下载CSV

动涡旋齿外侧型线方程:

(1)

\begin{array}{l} x_{m, o u} = a_{0} [(1 + c φ_{o u}^{δ_{1}}) c o s φ_{o u} + \\ (φ_{o u} + α_{o u} + \frac{c}{δ_{1} + 1} φ_{o u}^{δ_{1} + 1}) s i n φ_{o u}] \\ y_{m, o u} = a_{0} [(1 + c φ_{o u}^{δ_{1}}) s i n φ_{o u} - \\ (φ_{o u} + α_{o u} + \frac{c}{δ_{1} + 1} φ_{o u}^{δ_{1} + 1}) c o s φ_{o u}] \end{array}\}

式中: $c = δ_{0} / a_{0} .$

动涡旋齿内侧型线方程:

(2)

\begin{array}{l} x_{m, i n} = a_{0} [(1 + c φ_{i n}^{δ_{1}}) c o s (φ_{i n} + π) + \\ (φ_{i n} + α_{o u} + \frac{c}{δ_{1} + 1} φ_{i n}^{δ_{1} + 1} + \frac{R_{o b}}{a_{0}}) s i n (φ_{i n} + π)] \\ y_{m, i n} = a_{0} [(1 + c φ_{i n}^{δ_{1}}) s i n (φ_{i n} + π) - \\ (φ_{i n} + α_{o u} + \frac{c}{δ_{1} + 1} φ_{i n}^{δ_{1} + 1} + \frac{R_{o b}}{a_{0}}) c o s (φ_{i n} + π)] \end{array}\}

(3)

\begin{matrix} R_{o b} = a_{0} (π - α_{i n} - α_{o u}) + {(- 1)}^{δ_{1} + 2} \frac{δ_{0}}{δ_{1} + 1} (π - α_{i n} - α_{o u})^{δ_{1} + 1} \end{matrix}

根据表1所示基本几何结构参数构建的齿头修正变径基圆涡旋压缩机在吸气结束时刻的动、静涡旋齿啮合几何模型如图1(a)所示,工作腔从外到内依次为第3压缩腔、第2压缩腔及中心腔, 啮合三维模型如图1(b)所示.

图1

新窗口打开| 下载原图ZIP| 生成PPT

图1 动静涡旋齿几何模型

Fig.1 Schematic diagram of geometrical model of orbiting and fixed scroll teeth

由于变径基圆涡旋齿在不同展角处的基圆半径及齿厚都不相同,其齿头修正方法与普通圆渐开线涡旋齿齿头双圆弧修正有一定的差异,文献[3]中给出了其修正原理示意如图2(a)所示.图中:t₁, t₂,t₃为齿头部分3处齿厚的大小; R_in, R_ou为修正圆弧及连接圆弧半径; a_ou为渐开线展角Φ处对应的基圆半径;ρ_ou为外型线的曲率半径; γ, β,λ,d₁为修正角度及距离参数.在此基础上,利用面积分块法推导其修正齿面积如图2(b)所示.图中,S_bj为单个完整涡旋齿未修正部分面积.

图2

新窗口打开| 下载原图ZIP| 生成PPT

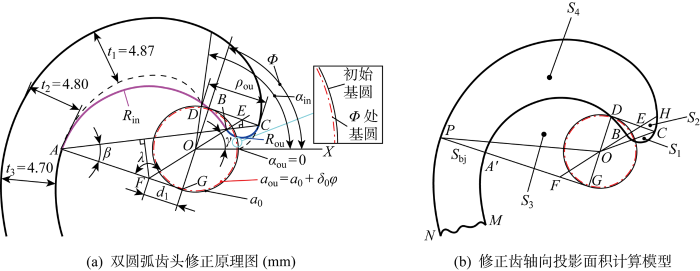
图2 双圆弧齿头修正原理及齿头面积计算模型

Fig.2 Modification principle and teeth head area calculation model of double-arc modification

修正齿轴向投影面积为

(4)

\begin{matrix} S_{m} = S_{1} + S_{2} - S_{3} + S_{4} \end{matrix}

式中:$S_1$为扇形BEC的面积; $S_3$为扇形A'FB的面积; $S_2$为渐开线扇形EHC面积; $S_4$为渐开线扇形OHPO与三角形POF的面积和.

1.2 变径基圆涡旋压缩机3种工作腔容积求解方法比较分析

准确且简便的涡旋压缩机工作腔容积变化规律是其几何模型及热动力学模型建立与求解的关键,目前常用的求解方法有法向等距法^[15]、图解法以及格林定理法^[16].利用格林定理不难推导封闭压缩工作腔的轴向投影面积为

(5)

\begin{array}{l} A (φ) = \int_{φ - 3 π}^{φ - π} \frac{1}{2} (x_{f, i n} \frac{d y_{f, i n}}{d φ_{i n}} - y_{f, i n} \frac{d x_{f, i n}}{d φ_{i n}}) d φ_{i n} - \\ \int_{φ - 3 π}^{φ - π} \frac{1}{2} (x'_{m, o u} \frac{d y'_{m, o u}}{d φ_{o u}} - y'_{m, o u} \frac{d x'_{m, o u}}{d φ_{o u}}) d φ_{o u} = \\ π R_{o b} [R_{o b} - 4 a_{0} π + 2 a_{0} φ + \frac{2}{3} \frac{δ_{0} (3 φ^{2} - 12 π φ + 13 π^{2})}{δ_{1} + 1}] \end{array}

式中: x_f,in, y_f,in为静涡旋齿内侧型线方程.

当φ_ou=φ_e时有:

(6)

\begin{matrix} \begin{array}{l} x'_{m, o u} = x_{m, o u} + R_{o b} c o s (φ_{o u} - 1.5 π) \\ y'_{m, o u} = y_{m, o u} + R_{o b} s i n (φ_{o u} - 1.5 π) \end{array}\} \end{matrix}

因此,单个吸气腔轴向投影面积计算公式为

(7)

\begin{matrix} A_{s} = A (φ_{e}) \end{matrix}

压缩腔投影面积随主轴转角θ的变化规律为

(8)

\begin{matrix} A (θ) = A (φ_{e} - θ) \end{matrix}

对表1所示涡旋压缩机的基本几何结构参数利用3种求解方法进行求解比较分析,其示意如图3所示.

图3

新窗口打开| 下载原图ZIP| 生成PPT

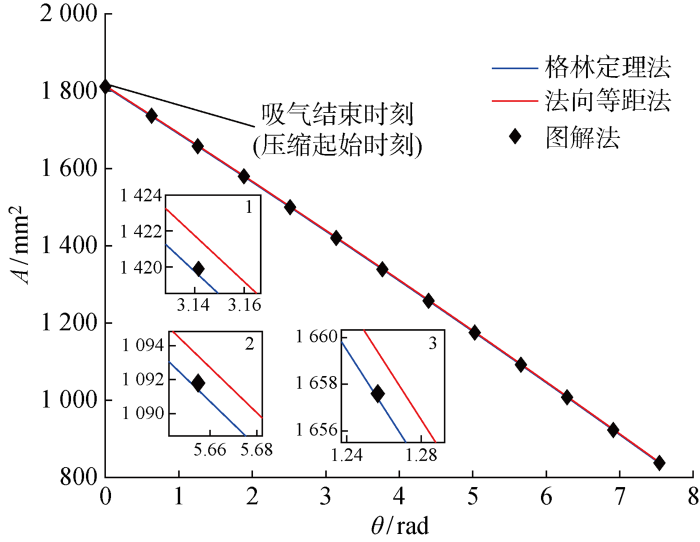
图3 不同容积求解方法计算结果对比

Fig.3 Comparison of calculation results of different working chamber volume solving methods

由图3可见,利用3种方法求解的压缩腔轴向投影面积变化规律高度一致.对3个不同转角位置(如图3中1, 2, 3)所示的曲线放大观察,格林定理法与图解法的误差较小而法向等距与其两者的误差相对较大,在这3个位置处格林定理法与图解法的误差分别为 0.036%、0.055%及 0.042 3%,主要原因是作图时的测量误差;法向等距法与图解法的误差分别为0.106%、0.128%及 0.078 4%.两者产生差异的原因是格林定理法的计算过程是直接利用生成变径基圆涡旋齿的型线参数方程进行代入求解,因此,误差相对较小;而法向等距法进行了几何等效处理,在平移组成封闭工作腔的内外型线时会造成微小的面积偏移误差,格林定理法虽然精度较高,但由于组成封闭工作腔的型线类型在不断变化,对于吸气过程及后续压缩过程面积的推导极其抽象复杂^[17].相反,法向等距法由于其简单易懂且精度满足要求,广泛应用于变壁厚涡旋压缩机的几何模型快速建立中.

对3组不同几何结构参数的变径基圆涡旋压缩机进行计算分析,研究其吸气结束时刻的单腔轴向投影面积的大小,计算结果如表2所示.表中几何参数1来源于表1,几何参数2和3分别来源于文献[18]和[19].

表2 单腔轴向投影面积

Tab.2 Axial projected area of single chamber mm²

计算方法	几何参数1	几何参数2^[18]	几何参数3^[19]
格林定理法	1 811.997 3	734.712 3	953.449 2
图解法	1 811.910 0	734.660 0	953.440 0
法向等距法	1 813.995 9	735.243 6	954.670 3

新窗口打开| 下载CSV

从表2可知,3组不同几何结构参数的变径基圆涡旋压缩机的吸气终了轴向投影面积大小满足图3所示的变化规律,再次证明了以上关于几何分析的正确性及通用性.

1.3 完整工作腔容积的变化规律

文献[15]中利用法向等距法给出了变径基圆涡旋压缩机压缩腔及排气腔容积的计算公式,对相应公式进行坐标变换及利用法向等距法推导出吸气腔的容积V₀,计算公式如下.

当0≤θ≤2π时,

(9)

\begin{matrix} V_{0} = 2 H R_{o b} {L_{0} + δ_{0} [(φ_{e} {- π - θ)}^{δ_{1}} - (φ_{e} {- π)}^{δ_{1}} - 54.789 1 s i n θ]} \end{matrix}

式中:H为齿高;L₀为基线长,

(10)

\begin{array}{l} L_{0} = \\ \int_{φ_{e} - π - θ}^{φ_{e} - π} a_{0} (φ + α_{o u} + \frac{δ_{0}}{a_{0}} \frac{1}{δ_{1} + 1} φ^{δ_{1} + 1} + \frac{R_{o b}}{2 a_{0}}) d φ \end{array}

行程容积为

(11)

\begin{matrix} V_{s} = 2 H A_{s} = V_{0} (2 π) \end{matrix}

式中:V₀(2π)为θ=2π时V₀的函数值.

理论内压缩比为

(12)

\begin{matrix} ε = {(\frac{V_{s}}{V (θ_{d})})}^{k} \end{matrix}

式中:$θ_d$为开始排气角; $V(θ_d) $为$θ=θ_d$时完整工作腔容积V的函数值;k为绝热指数.

由于涡旋压缩机的实际动、静涡旋盘是沿着齿高(轴向)方向进行拉伸生成的,所以工作腔容积及涡旋齿体积的计算为相应几何体的轴向投影面积与齿高的乘积,为了使得计算量减小,令H取值为 1 mm,但不影响变径基圆涡旋压缩机工作腔容积及相应几何模型变化规律的趋势及精确程度.利用MATLAB数值计算软件对表1所示参数的变径基圆涡旋压缩机进行编程计算,得到其从吸气开始到排气结束的完整工作腔容积V变化规律如图4所示.

图4

新窗口打开| 下载原图ZIP| 生成PPT

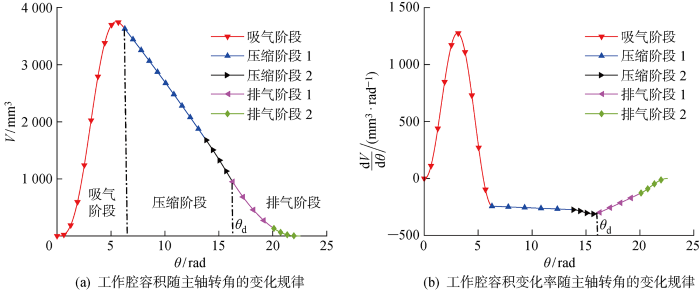
图4 工作腔容积及其变化率变化规律

Fig.4 Evolution and derivative of working chamber volume with orbiting angle

图4(a)所示为变径基圆涡旋压缩机完整工作腔容积随主轴转角的变化规律.同其他类型涡旋压缩机一致,在吸气阶段,工作腔容积逐渐增大至峰值然后小幅度的减小直到吸气腔完全闭合,随着压缩阶段的逐渐进行,工作腔容积持续减小直到动静涡旋齿达到最终啮合点即主轴转角为θ_d时,第2压缩腔与中心腔相连通进入排气阶段,持续到排气腔容积减小为0,排气过程结束.图4(b)所示为工作腔容积的变化率随着主轴转角的变化规律.由图可知,各个阶段工作腔容积的变化速率存在明显差异,吸气阶段,工作腔容积先增加较快,当dV/dθ=0时,吸气腔容积达到最大,随后,dV/dθ<0,工作腔容积逐渐减小一直到排气过程结束.

1.4 齿面积利用率计算模型

由于变径基圆涡旋压缩机的涡旋齿壁厚逐渐变化,属于渐变壁厚涡旋类型,且涡旋齿的面积利用率能够衡量其材料的使用率^[20-21],同时也能间接反映工作腔轴向投影面积的大小及汽车空调用涡旋压缩机轻量化的要求,齿面积利用率越小,耗材越少,质量越小.定义齿面积利用率ζ为动静涡旋齿的齿面积和占两者啮合所需最小盘面积的大小,计算示意如图5所示.

图5

新窗口打开| 下载原图ZIP| 生成PPT

图5 齿面积利用率计算模型

Fig.5 Calculation model of area utilization rate

动、静涡旋齿啮合所需最小盘径^[3]:

(13)

\begin{matrix} D_{m i n} = 2 (a_{0} φ_{e} + \frac{δ_{0}}{δ_{1} + 1} φ_{e}^{δ_{1} + 1} + R_{o b}) \end{matrix}

动、静涡旋齿面积:

(14)

S_{o} = S_{f} = S_{x}

(15)

\begin{matrix} S_{x} = S_{b j} + S_{m} \end{matrix}

式中:S_m为齿头采用双圆弧修正的修正齿轴向投影面积.

因此,齿面积利用率为

ζ = \frac{8 S_{x}}{π D_{m i n}^{2}}

不同初始基圆半径a₀对应的齿面积利用率随基圆半径变化系数δ₀的变化如图5(b)所示.从图中可知,随着$δ_0$的增大,齿面积利用率均呈非线性的增大趋势,且增大速率越来越慢.不同基圆半径对应的变化规律曲线汇聚于$δ_0=0$点, $δ_0<0$时, $ζ$与$a_0 $成正比; $δ_0>0$时, $ζ$与$a_0$成反比.同理,随着$α_{in}$的增大,ζ也相应增大.因此,合理地选择型线参数对齿面积利用率指标有着重要影响.

2 型线参数对齿面积利用率及行程容积的影响

为了研究各型线参数对变径基圆涡旋压缩机几何性能的影响程度大小,为后续的优化分析确定最佳的决策变量,定义行程容积与齿面积利用率的灵敏度模型为

(16)

\begin{matrix} S_{V_{s}} = \frac{\partial V_{s}}{\partial X_{i}} \end{matrix}

(17)

\begin{array}{l} S_{ζ} = \frac{\partial ζ}{\partial X_{i}} \end{array}

式中: $X_{i} = [x_{1} x_{2} x_{3} x_{4} x_{5} x_{6}]^{T} = [a_{0} δ_{0} α_{i n} Φ φ_{e} {H]}^{T} .$

行程容积及齿面积利用率的灵敏度模型如图6所示,从图6(a)可知,$a_0$,$δ_0$,,H与行程容积之间为正比关系,$α_in$与其为反比关系,修正展角Φ对其几乎没有影响;从图6(b)可知,$a_0$,$δ_0$,$α_{in}$与齿面积利用率之间为正比关系,而Φ,$φ_e$,H对其几乎不产生影响, $δ_0$,$α_{in}$对两种几何性能的影响远大于其他参数,且基圆半径变化系数的影响最大.

图6

新窗口打开| 下载原图ZIP| 生成PPT

图6 灵敏度分析模型

Fig.6 Model of sensitivity analysis

3 多目标优化分析模型的建立与求解

基于建立的变径基圆涡旋压缩机几何模型,对某款汽车空调用变基圆半径渐开线涡旋压缩机^[18]几何结构参数及性能进行优化分析,比较不同优化算法求解性能指标的差异.

3.1 目标函数及决策变量的确定

从上述分析知,$δ_0$,$α_{in}$对行程容积及齿面积利用率的影响尤其显著,且$V_s$与$ζ$之间不能同时取得最优,存在明显的冲突关系.因此,选取$V_s$ 与$ζ$为优化目标, $δ_0$,$α_{in}$为优化决策变量.

3.2 约束条件的确定

(1) 变径基圆涡旋压缩机常用于汽车空调等领域^[11].因此,动静涡旋齿的最小啮合盘径定为

(18)

\begin{matrix} 80 m m \leq D_{m i n} \leq 150 m m \end{matrix}

(2) 采用法向等距法生成涡旋齿,回转半径大小会影响齿厚大小及内外型线位置.因此,将其定为

(19)

\begin{matrix} a_{0} \leq R_{o b} \leq 2 a_{0} \end{matrix}

(3) 为了使得优化分析有意义,须保证优化分析之后的内压缩比不小于优化前的内压缩比.因此,将其定为

(20)

\begin{matrix} ε_{0} \geq ε \end{matrix}

(4) 考虑到变径基圆涡旋压缩机在φ_e处涡旋齿的强度及刚度,将该处齿厚t(φ_e)定为

(21)

\begin{array}{l} 1 m m \leq t (φ_{e}) = [(x_{m, i n} (φ_{e} - π) - \\ x_{m, o u} (φ_{e} {))}^{2} + (y_{m, i n} (φ_{e} - π) - \\ y_{m, o u} (φ_{e} {))}^{2}]^{1 / 2} \leq 3 m m \end{array}

(5) 为了使得涡旋压缩机的壁厚变化规律适应工质在工作腔内压力、温度向中心腔靠近逐渐增大的变化规律,将基圆半径变化系数定为

(22)

\begin{matrix} - 1 \leq δ_{0} \leq 0 \end{matrix}

3.3 求解结果及比较分析

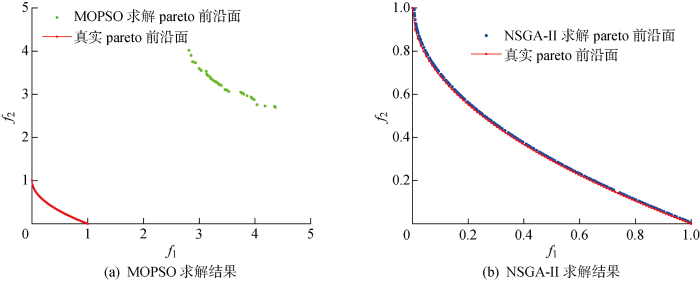
多目标优化算法与传统的线性加权法相比,能够把可供决策者选取的多组解以非劣解集的形式可视化,更具优势.多目标遗传算法(NSGA-II)及多目标粒子群(MOPSO)算法是在传统遗传算法及粒子群算法的基础上,加入了非支配排序算子,超体积(HV)、反世代距离(IGD)、计算时间(T)能够衡量算法的优劣,HV值越大,IGD值越小,时间越短,算法越优秀.表3所示为以上两种算法求解标准测试函数BT1的相关性能指标对比.从表3可知,在相同种群数量及迭代次数的条件下,NSGA-II在计算时间、HV及IGD指标均优于MOPSO.图7所示为多目标优化算法NSGA-II及MOPSO求解标准测试函数BT1(f₁, f₂为其两个目标函数)的结果.由图可见,MOPSO求解^[22]获得的pareto前沿均匀性及准确性均差于NSGA-II对应的求解结果.因此,对于测试函数BT1, NSGA-II更具优势.图8(a)所示为只考虑δ₀,α_in上下边界约束条件的求解结果,由图可知,对于较少的决策变量,MOPSO与NSGA-II能解得差异极小的非劣解集,但MOPSO对应解的分布均匀性弱于NSGA-II, 且计算时间为12.70 s,多于11.11 s.图8(b)为考虑非线性约束条件的求解结果,其计算时间为25.56 s,虽计算效率弱于图8(a)中的结果,但求解的非劣解集更精确,范围更小,决策者选取优秀的解也更容易.从图8(b)中选取一组非劣解及其对应的型线参数与优化前作对比,如表4所示.

图7

新窗口打开| 下载原图ZIP| 生成PPT

图7 测试函数求解结果

Fig.7 Solutions to test function

图8

新窗口打开| 下载原图ZIP| 生成PPT

图8 优化分析模型求解结果

Fig.8 Solutions to optimization analysis model

表3 性能指标对比

Tab.3 Comparison of performance indicators

算法名称	种群大小	迭代次数	T/s	HV	IGD
NSGA-II	200	800	4.81	0.712 4	0.008 032
MOPSO	200	800	5.19	0	3.888 300

新窗口打开| 下载CSV

由表4可知,优化分析之后的优化型在满足内压比的条件下,较优化前,行程容积增大了6.95%,齿面积利用率下降了4.26%,证明了本优化分析模型的实用性.同时,在本优化分析模型及测试函数上,NSGA-II综合性能均优于MOPSO.

表4 优化型与优化前几何性能对比

Tab.4 Comparison of geometric performance of optimized and no-optimized type

名称	δ₀	α_in/rad	V_s×10⁵/m³	ζ	ε
优化前	-0.020 0	1.317 8	2.316	0.276 7	4.315 6
优化型	-0.014 3	1.222 0	2.477	0.264 9	4.343 6

新窗口打开| 下载CSV

4 结语

完善了变径基圆涡旋压缩机的几何模型,给出了其完整工作过程的容积变化规律,讨论了格林定理法、图解法及法向等距法计算结果的差异性,3种方法的计算结果误差小,均可用于变径基圆涡旋压缩机几何模型的求解,但法向等距法更简单易懂.建立了变径基圆涡旋压缩机齿面积利用率的计算模型,分析了各几何结构参数对行程容积及齿面积利用率的影响程度,发现基圆半径变化系数及内侧型线发生角的影响尤为显著.构建了一种以V_s与ζ为优化目标的多目标优化分析模型,优化分析之后的性能均优于优化前,且优化算法NSGA-II较MOPSO更具优势,该模型能为变径基圆涡旋压缩机的优化研究提供进一步的理论参考.

参考文献

原文顺序

文献年度倒序

文中引用次数倒序

被引期刊影响因子

[1]

刘振全.

涡旋式流体机械与涡旋压缩机[M]. 北京: 机械工业出版社, 2009.